Formula For Area Of A Circle With Circumference

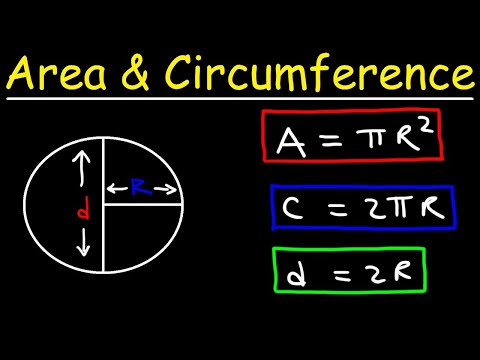
The area of the circle can be conveniently calculated either from the radius, diameter, or circumference of the circle. The constant used in the calculation of the area of a circle is pi, and it has a fractional numeric value of 22/7 or a decimal value of 3.14. Any of the values of pi can be used based on the requirement and the need of the equations.

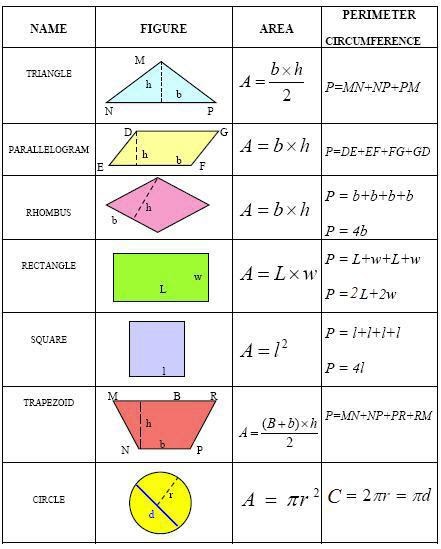
The below table shows the list of formulae if we know the radius, the diameter, or the circumference of a circle. As with triangles and rectangles, we can attempt to derive formulas for the area and "perimeter" of a circle. Calculating the circumference of a circle is not as easy as calculating the perimeter of a rectangle or triangle, however. Given an object in real life having the shape of a circle, one approach might be to wrap a string exactly once around the object and then straighten the string and measure its length. Diameter is the line that separates the circle into two equal parts and is also equal to twice the radius.

A circle is a fundamental shape that is measured in terms of its radius. In geometry or mathematics, a circle can be defined as a special variety of ellipse in which the eccentricity is zero and the two foci are coincident. The area of a circle formula is useful for measuring the region occupied by a circular field or a plot. Suppose, if you have a circular table, then the area formula will help us to know how much cloth is needed to cover it completely.

The area formula will also help us to know the boundary length i.e., the circumference of the circle. A circle is a two-dimensional shape, it does not have volume. A circle only has an area and perimeter/circumference.

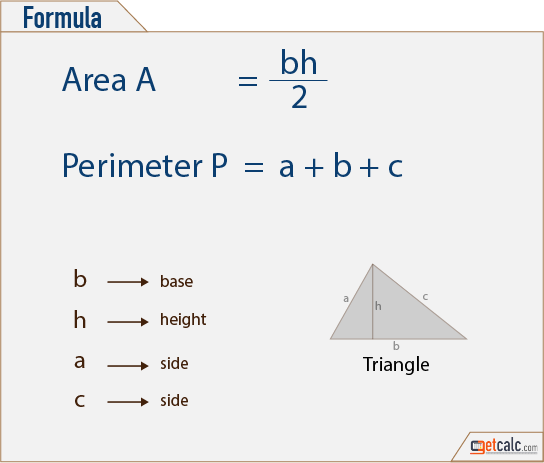
Let us learn in detail about the area of a circle, surface area, and its circumference with examples. The first solution requires a general understanding of similarity of shapes while the second requires knowledge of similarity specific to triangles. The circumference of a circle of radius $r$ is $2\pi r$. This well known formula is taken up here from the point of view of similarity.

It is important to note in this task that the definition of $\pi$ already involves the circumference of a circle, a particular circle. In order to show that the ratio of circumference to diameter does not depend on the size of the circle, a similarity argument is required. Two different approaches are provided, one using the fact that all circles are similar and a second using similar triangles. This former approach is simpler but the latter has the advantage of leading into an argument for calculating the area of a circle.

A circle is a closed shape formed by tracing a point that moves in a plane such that its distance from a given point is constant. The word circle is derived from the Greek word kirkos, meaning hoop or ring. In this article, we cover the important terms related to circles, their properties, and various circle formulas. This first argument is an example of MP7, Look For and Make Use of Structure. The key to this argument is identifying that all circles are similar and then applying the meaning of similarity to the circumference.

The second argument exemplifies MP8, Look For and Express Regularity in Repeated Reasoning. Here the key is to compare the circle to a more familiar shape, the triangle. The perimeter and area of triangles, quadrilaterals , circles, arcs, sectors and composite shapes can all be calculated using relevant formulae. In above program, we first take radius of circle as input from user and store it in variable radius.

Then we calculate the circumference of circle using above mentioned formulae and print it on screen using cout. A circle is known as a closed plane geometrical shape. Technically, it is the locus of a point that moves around a fixed point at a fixed distance that is away from that point. A circle is basically a closed curve that has its outer line at an equal distance from the centre.

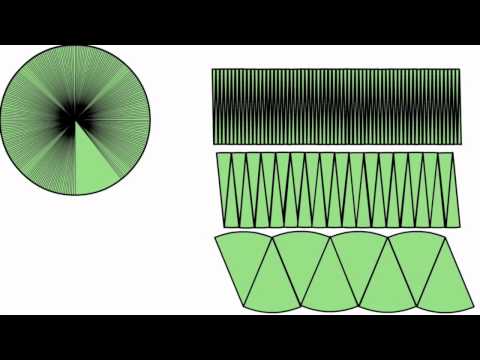
This fixed distance from the central point is known as the radius of the circle. In our day to day life, we often see many examples like a pizza, wheel, etc. Let us learn about these terms in regards to a circle. Cover the circle with concentric circles of "r" radius. After dividing the circle along the designated line shown in the above figure and spreading the lines, the outcome will be a triangle. The base of the triangle will be equivalent to the circumference of the circle, and its height will be identical to the radius of the circle.

The distance from the centre to the outer line of the circle is called a radius. It is the most important quantity of the circle based on which formulas for the area and circumference of the circle are derived. Twice the radius of a circle is called the diameter of the circle. The diameter cuts the circle into two equal parts, which is called a semi-circle. A circle can be divided into many small sectors which can then be rearranged accordingly to form a parallelogram.

When the circle is divided into even smaller sectors, it gradually becomes the shape of a rectangle. We can clearly see that one of the sides of the rectangle will be the radius and the other will be half the length of the circumference, i.e, π. As we know that the area of a rectangle is its length multiplied by the breadth which is π multiplied to 'r'. When we have the length of the diameter or the radius or the circumference of the circle, we can find the surface area of the circle by using the surface area formula.

This surface area of the circle is represented in terms of square units. The perimeter of a closed figure is known to be the length of its total boundary. When it comes to the circles, the perimeter is called by a different name.

It is referred to as the 'circumference' of the given circle. This circumference is known as the total length of the boundary of the given circle. If we open the circle and form a straight line, the length of the straight line that we get is the circumference. For defining the circumference of a circle, we need to know a term called 'pi'. Consider the circle shown below having its centre at O and radius r. We use this formula to measure the space which is occupied by either a circular plot or a field.

In this article today, we will discuss the area of circle definition, the area of a circle equation, its circumference and surface area in detail. The sectors are pulled out of the circle and are arranged as shown in the middle diagram. The length across the top is half of the circumference. When placed in these positions, the sectors form a parallelogram. The larger the number of sectors that are cut, the less curvy the arcs will appear and the more the shape will resemble a parallelogram.

As seen in the last diagram, the parallelogram ca be changed into a rectangle by slicing half of the last sector and placing it to the far left. I am going to discuss these axioms in a moment, but first let me show how Claim follows. But by Axiom 1 the length of the arc $PBQ$ is greater than $PQ$, while it follows from Euclid III.2 that $OB$ is greater than $OA$. Applying symmetry, this implies Claim in this case, and ought to suggest how the reasoning goes in general. For those having difficulty using formulas manually to find the area, circumference, radius and diameter of a circle, this circle calculator is just for you.

The equations will be given below so you can see how the calculator obtains the values, but all you have to do is input the basic information. Like all geometric shapes, circles take up space and a formula is required to calculate the area. Say you're trying to calculate the area of a circle with a radius of 3 inches. You would multiply 3 times 3 to get 9, and multiply 9 times π. Also note that when you multiply inches by inches, you get square inches, which is a measurement of area instead of length. Calculating either the circumference or area of a circle requires knowing the circle's radius.

A circle's radius is the distance from the center of the circle to any point on the edge of the circle. Radius is the same for all points on a circle's edge. One of your problems might give you diameter instead of radius and ask you to solve for area or circumference. A circle's diameter is equal to the distance across the center of the circle, and is equal to the radius times 2. So, you can convert diameter to radius by dividing the diameter by 2. For example, a circle with a diameter of 8 has a radius of 4.

The diameter of the circle is double the radius of the circle. Hence the area of the circle formula using the diameter is equal to π/4 times the square of the diameter of the circle. The formula for the area of the circle, using the diameter of the circle π/4 × diameter2.

Write the formula for circumference of circle with radius r. Is called the circumference and is the linear distance around the edge of a circle. The circumference of a circle is proportional to its diameter, d, and its radius, r, and relates to the famous mathematical constant, pi (π). When you are doing calculations involving a circle, you frequently use the number π, or pi. Pi is defined as being equal to the circumference of a circle -- the distance around that circle -- divided by its diameter.

However, you don't need to memorize this formula when working with π, since it is a constant. Students beginning geometry can expect to encounter problem sets that involve calculating the area and circumference of a circle. You can solve these problems as long as you know the circle's radius and can do some simple multiplication. If you learn the value of the constant π and the basic equations for a circle's properties, you can quickly find the area or circumference of any circle.

If the area of the circle is not equal to that of the triangle, then it must be either greater or less. We eliminate each of these by contradiction, leaving equality as the only possibility. The area of a regular polygon is half its perimeter times the apothem. As the number of sides of the regular polygon increases, the polygon tends to a circle, and the apothem tends to the radius. This suggests that the area of a disk is half the circumference of its bounding circle times the radius. A circle is a collection of points that are at a fixed distance from the center of the circle.

We see circles in everyday life such as a wheel, pizzas, a circular ground, etc. The measure of the space or region enclosed inside the circle is known as the area of the circle. The perimeter of the circle equals the length of its total boundary.

The length of the rope that wraps around its boundary is equal to its circumference. We can measure this by using the formula given below. And as Math Open Reference states, the formula takes the circumference of the entire circle (2πr). It reduces it by the ratio of the degree measure of the arc angle to the degree measure of the entire circle . To recall, the area is the region that occupied the shape in a two-dimensional plane.

In this article, you will learn the area of a circle and the formulas for calculating the area of a circle. From point B, on the circle, draw another circle with center at B, and radius OB. The intersections of the two circles at A and E form equilateral triangles AOB and EOB, since they are composed of 3 congruent radii. Extend the radii forming these triangles through circle O to form the inscribed regular hexagon with 6 equilateral triangles.

In this formula, r represents the radius, which is a segment that connects the center of the circle to a point on the edge of the circle. It is half the size of the diameter, and every radius inside of a circle will be the same. Let's take a brief look at how to find the area of circles.

Π shows the ratio of the perimeter of a circle to the diameter. Therefore, when you divide the circumference by the diameter for any circle, you obtain a value close enough to π. This relationship can be explained by the formula mentioned below.

When we use the formula to calculate the circumference of the circle, then the radius of the circle is taken into account. Hence, we need to know the value of the radius or the diameter to evaluate the perimeter of the circle. So we can use the area of a circle formula to calculate the area of the pizza. Observe the above figure carefully, if we split up the circle into smaller sections and arrange them systematically it forms a shape of a parallelogram. The more the number of sections it has more it tends to have a shape of a rectangle as shown above. Then we calculate the area of circle using above mentioned formulae and print it on screen using cout.

The area of circle is the amount of two-dimensional space taken up by a circle. We can calculate the area of a circle if you know its radius. Write the formula of area, making r as the subject. The area of a circle is the total number of square units that fill the circle. The area of the following circle is about 13 units. Note that we count fractional units inside the circle as well as whole units.

Angles formed by the same arc on the circumference of the circle is always equal. In this method, the circle is divided into many small sectors and the arrangement of the sectors has been done in the form of a parallelogram as shown in the figure below. Clearly, if the circle is divided into more sectors, the parallelogram will turn into a rectangle. Any given geometrical shape possesses its own area. This area is referred to as the region which is occupied by the shape in a 2D plane.